Question

Suppose there is a 14.3% probability that a randomly selected person aged 25 years or older is a In​ addition, there is a probability that a randomly selected person aged years or older is given that he or she What is the probability that a randomly selected person aged years or older is and Would it be unusual to randomly select a person aged years or older who is and

Answer 1

Complete Question

Suppose there is a 14.3% probability that a randomly selected person aged 25 years or older is a jogger. In addition, there is a 52.6% probability that a randomly selected person aged 25 years or older is female, given that he or she jogs. What is the probability that a randomly selected person aged 25 years or older is female and jogs? Would it be unusual to randomly select a person aged 25 years or older who is female and jogs?

Answer:

The probability is
`P(F \ n \ J ) = 0.075`

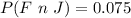
No it is not unusual

Explanation:

From the question we are told that

The probability that a randomly selected person aged 25 or older is a jogger is

`P(J) = 0.143`

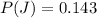
The probability that a randomly selected person aged 25 years or older is female, given that he or she jogs

`P(F | J) = 0.526`

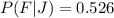
Generally the the probability that a randomly selected person aged 25 years or older is female and jogs is mathematically evaluated using Bayes rules as follows

`P(F \ n \ J ) = P(F | J ) * P(J )`

=>
`P(F \ n \ J ) = 0.526 * 0.143`

=>
`P(F \ n \ J ) = 0.075`

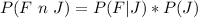
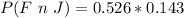
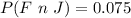
Generally this is not unusual because value obtained is greater than 0.05