Question

31 32 33 34 35 36 37 38 39 40

Sophia’s math test is worth 130 points and has 40 problems. Each problem is worth either 4 points or 3 points. How many of each question type were on the test?
The test had 30 4-point questions and 10 3-point questions.
The test had 10 4-point questions and 30 3-point questions.
The test had 28 4-point questions and 12 3-point questions.
The test had 25 4-point questions and 10 3-point questions.

Answer 1

B.

Explanation:

Answer 2

The correct answer is: The test had 10 4-point questions and 30 3-point questions.

Explanation:

Let t be the number of 3-point questions on the test

and

f be the number of 4-point questions on the test

Then,

According to the given statement that the test had 40 problems

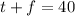`t+f = 40` Eqn 1

And

According to given statement that the total points are 130

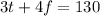`3t+4f = 130` Eqn 2

These equations form a system of linear equations in two variables.

Elimination method or substitution method can be used to solve the system.

So, from equation 1:

`t+f = 40\\t = 40-f`

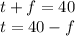
Putting t = 40-f in equation 2

`3(40-f) +4f = 130\\120-3f+4f = 130\\`

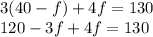
Subtracting 120 from both sides

`120-3f+4f-120 = 130-120\\f = 10`

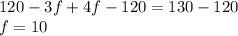
Putting f = 10 in equation 1

`t+10 = 40`

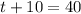
Subtracting 10 from both sides

`t+10-10 = 40-10\\t = 30`

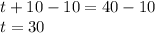
Hence,

Number of 3-point questions = t = 30

Number of 4-point questions = f = 10

So,

The correct answer is: The test had 10 4-point questions and 30 3-point questions.