Question

*PLS HELP*

I need help on this and please provide an explanation
Thank you!

Question: What is the value of X°
In this complementary angle? ​

Answer 1

x=30

Yes, they are complementary

Explanation:

The two angles shown add up to the right angle marked. Right angles are 90 degrees. Set up the following equation:

2x°+x°=90

3x°=90

x=30

Complementary angles are two angles that add up to 90°. Since the two angles add up to 90 degrees, they are complementary.

Answer 2

`\boxed{\boxed{ \tt \: x = 30 {}^( \circ)}}`

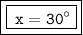
Explanation:

The Given two angles are complementary angles .

The Two angles are 2x° and x°.[Given]

[Two angles are called complementary if their sum is 90°. Each angle is a complement to each other.]

We need to find the value of x.

So,

`\tt2x {}^( \circ) + {x}^( \circ) = 90{}^( \circ)`

Solve this equation.

`\tt \implies(2x + {x} ){}^( \circ) = 90{}^( \circ)`

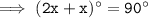
Combine the like terms:

`\tt3x{}^( \circ) = 90{}^( \circ)`

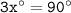
Divide both sides by 3 :

`\tt \implies \cfrac{3x{}^( \circ) }{3{}^( \circ) } = \cfrac{90{}^( \circ) }{3{}^( \circ) }`

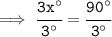
Cancel the LHS and RHS:

`\tt \implies \cfrac{ \cancel{3x{}^( \circ)} }{ \cancel{3{}^( \circ)} } = \cfrac{ \cancel{90}{}^( \circ) }{ \cancel{3{}^( \circ)} }`

`\tt \implies \cfrac{1x{}^( \circ) }{1{}^( \circ) } = \cfrac{30{}^( \circ) }{1{}^( \circ) }`

`\tt \implies 1x{}^( \circ) = 30{}^( \circ)`

`\tt \implies x{}^( \circ) = 30{}^( \circ)`

`\tt \implies x{}^( \circ) = 30{}^( \circ)`

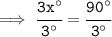
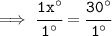
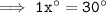
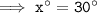
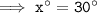
Hence, the value of x° would be 30°.

`\rule{225pt}{2pt}`

I hope this helps!