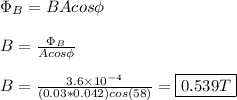Question

A horizontal rectangular surface has dimensions 3.00 cm by 4.20 cm and is in a uniform magnetic field that is directed at an angle of 32.0 ∘ above the horizontal.

What must the magnitude of the magnetic field be to produce a flux of 3.60 × 10 −4 Wb through the surface?

Answer 1

Hi there!

Recall the equation for magnetic flux.

`\Phi _B = \oint B \cdot dA`

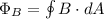
We can also rewrite this as:

`\Phi_B = B \cdot A = BAcos\phi`

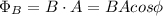
B = Magnetic field strength (T)
A = Area of surface (m²)

φ = angle between the surface's area vector and magnetic field

Since the rectangular surface is horizontal, its perpendicular area vector points straight UP.

The magnetic field makes an angle of 32° with the surface but makes an angle complementary to this angle with the AREA VECTOR. (Think: the vertical)

Complementary of 32° ⇒ 90° - 32° = 58°

Now, we can rearrange the above equation to solve for the magnetic field.

`\Phi_B = BA cos\phi \\\\B = (\Phi_B)/(Acos\phi)\\\\B = (3.6 * 10^(-4))/((0.03 * 0.042)cos(58)) = \boxed{0.539 T}`