Question

A string under a tension of 53.9 N is used to whirl a rock in a horizontal circle of radius 2.59 m at a speed of 21.8 m/s. The string is pulled in and the speed of the rock increases. When the string is 1.23 m long and the speed of the rock is 54.8 m/s, the string breaks. What is the breaking strength (in newtons) of the string

Answer 1

The breaking strength of the string is 717.19 N

Step-by-step explanation:

Given;

initial tension on the string, T₁ = 53.9 N

initial radius of the string, r₁ = 2.59 m

initial speed of the rock, v₁ = 21.8 m/s

final radius of the string, r₂ = 1.23 m

final speed of the rock, v₂ = 54.8 m/s

the final tension on the string = breaking strength, T₂ = ?

Apply centripetal force equation due to inward motion;

`T= (mv^2)/(r)\\\\Tr = mv^2\\\\m =(Tr)/(v^2)\\\\ (T_1r_1)/(v_1^2) = (T_2r_2)/(v_2^2)\\\\T_2 = (T_1r_1v_2^2)/(r_2v_1^2)\\\\ T_2 = ((53.9)(2.59)(54.8)^2)/((1.23)(21.8)^2)\\\\ T_2 = 717.19 \ N`

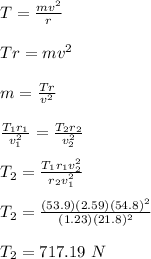
Therefore, the breaking strength of the string is 717.19 N