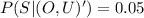Question

Five different companies (G, J, S, O, U) compete each year to be the sole supplier of relays to a major automobile manufacturer. Supplier G J S O U probability .15 .04 .01 .55 .25 Given that suppliers O and U are both ultimately not chosen, what is the probability that supplier S will be chosen

Answer 1

The probability is
`P(S | (O ,U)' ) = 0.05`

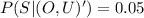
Explanation:

From the question we are told that

The probability of each company to be chosen is

Supplier G J S O U

Probability 0.15 0.04 0.01 0.55 0.25

Generally the probability that company G , J , S are chosen is

`P(G , J , S ) = 0.15 + 0.04 + 0.01`

=>
`P(G , J , S ) = 0.20`

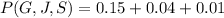
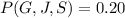
Generally the probability that company O, U are chosen is

`P(O, U ) = 0.55+ 0.25`

=>
`P(O, U ) = 0.80`

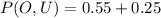
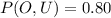
Generally the probability that supplier S will be chosen given that suppliers O and U are both ultimately not chosen is mathematically represented as

`P(S | (O ,U)' ) = ( P(S \ n \ (O , U)' ))/( P((O, U)'))`

`P((O, U)' = 1 - P(O , U)`

`P((O, U)' = 1 - 0.8`

`P((O, U)' =0.2`

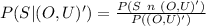
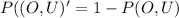
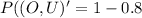
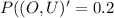
And

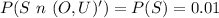
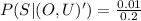
`P(S \ n \ (O , U)' ) = P(S) = 0.01`

=>
`P(S | (O ,U)' ) = ( 0.01 )/( 0.2)`

=>
`P(S | (O ,U)' ) = 0.05`