Question

Write an equation for the translation of y=6/x that has the asymptotes x = 4 and y = 5

Answer 1

`\displaystyle y=(30x)/(6(x-4))`

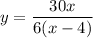
Explanation:

Keep in mind that a rational function with a vertical asymptote at
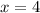`x=4` means the denominator must be 0 when
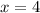`x=4` is plugged in.

This means we have
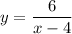`\displaystyle y=(6)/(x-4)` so far.

To account for the horizontal asymptote at
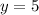`y=5`, we need to adjust the numerator and denominator so that they are the same degree and that the leading coefficients have a ratio of 5. We can do this by multiplying the numerator by 5x and the denominator by 6.

This leaves us with
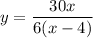`\displaystyle y=(30x)/(6(x-4))` as the translated equation since the end behavior of the function is
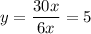`\displaystyle y=(30x)/(6x)=5`. See attached graph.