Answer:
The value is

Step-by-step explanation:
From the question we are told that
The mass of the crate is
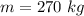
The force applied by the first worker is

The force applied by the second worker is

Generally the kinetic frictional force between the crate and the floor is equal to the force applied by both worker , this is mathematically represented as

Here
 is the frictional force and this is mathematically represented as
is the frictional force and this is mathematically represented as
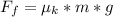
So
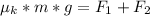
=>
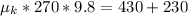
=>
