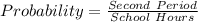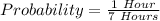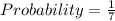Question

PLEASE EXPLAIN HOW YOU GOT ANSWER. A school administrator tells everyone in the building that they will be having a fire drill randomly sometime during the school day which is from 8 a.m. to 3 p.m. If second period is from 9:00 a.m. to 10:00 a.m., what is the probability of the fire drill happening during second period?

Answer 1

Answer: 14.3%

Step-by-step explanation: 1/7=14.3%

Answer 2

`Probability = (1)/(7)`

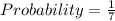
Explanation:

Given

`School\ Hours = 8am\ to\ 3pm`

`Second\ Period = 9am\ to\ 10am`

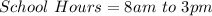
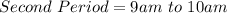
Required

Determine the probability that the fire drill happens in the second period

First, we need to convert both time range to hours.

8 am to 3 pm is 7 hours.

So:

`School\ Hours = 7\ hours`

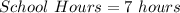
9 am to 10 am is 1 hour.

So:

`Second\ Period = 1\ hour`

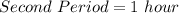
The required probability can then be calculated using:

`Probability = (Required\ Events)/(Total\ Events)`

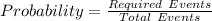
In this case, it is:

`Probability = (Second\ Period)/(School\ Hours)`

`Probability = (1\ Hour)/(7\ Hours)`

`Probability = (1)/(7)`