Question

Find the length of d in the figure below if a = 8 in., b = 3 in., and c = 4 in. Round your answer to the nearest tenth. Pls help :(

Answer 1

Length of side 'd' = 9.4 in.

Explanation:

From the picture attached,

Length of the sides of the given prism,

a = 8 in.

b = 3 in.

c = 4 in.

We have to find the measure of side 'd'.

By applying Pythagoras theorem in right triangle ABC,

AC² = AB² + BC²

AC =
`√(AB^2+BC^2)`

=
`√(a^2+b^2)`

=
`√(8^2+3^2)`

=
`√(73)`

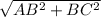
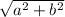
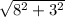
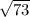
Now we apply Pythagoras theorem in right triagle ΔACE,

AE² = AC² + EC²

d² =
`(√(73))^2` + c²

d² = 73 + (4)²

d² = 89

d = √89

= 9.43

≈ 9.4 in.

Therefore, length of side 'd' is 9.4 in.