Question

The first three terms of an arithmetic progression are 2x, x+4, 2x - 7 respectively.

a. Find the value of x
b. Find the three terms
(6 m
c. What is the common difference?

Answer 1

a) x = 7.5

b) 15, 11.5, 8

c) -3.5

Explanation:

As there is a common difference between consecutive terms of an arithmetic progression, then:

`a_3-a_2=a_2-a_1`

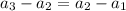
Given:

• 
  `a_1=2x`
• 
  `a_2=x+4`
• 
  `a_3=2x-7`

Therefore:

`\implies a_3-a_2=a_2-a_1`

`\implies (2x-7)-(x+4)=(x+4)-2x`

`\implies 2x-7-x-4=x+4-2x`

`\implies x-11=-x+4`

`\implies 2x=15`

`\implies x=7.5`

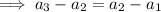
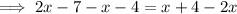
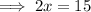
Inputting the found value of x into the term expressions to find the three terms of the arithmetic progression:

• 
  `a_1=2(7.5)=15`
• 
  `a_2=(7.5)+4=11.5`
• 
  `a_3=2(7.5)-7=8`

The common difference (d) is the difference between each consecutive term. To find the common difference, subtract one term from the next term:

`\implies d=a_3-a_2= 8 - 11.5 = -3.5`

`\implies d=a_2-a_1= 11.5-15 = -3.5`

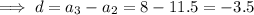
Therefore, the common difference is -3.5