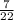The probability of picking a blue marble and flipping heads is
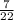
To find the probability of two independent events occurring, you can multiply the probabilities of each event.
Let's denote:
Event A: Picking a blue marble
Event B: Flipping heads
The probability of picking a blue marble (Event A) is the number of favorable outcomes (blue marbles) divided by the total number of outcomes (total marbles).
P(A)=
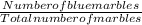
P(A)=

Now, the probability of flipping heads (Event B) is 1/2, assuming a fair coin.
P(B)=

To find the probability of both events happening, you multiply these probabilities:
P(A and B)=P(A)×P(B)
P(Blue marble and Heads)=
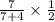
Now, calculate the product:
P(Blue marble and Heads)=
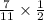
Multiplying the numerators and denominator
P(Blue marble and Heads)=
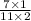
P(Blue marble and Heads)=
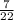
So, the probability of picking a blue marble and flipping heads is