Answer:
D.
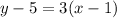
B.
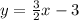
Step-by-step explanation:
Problem 1:
Point-slope form equation is given as
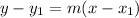 , where, (x1, y1) is a point on the line, and m = slope.
, where, (x1, y1) is a point on the line, and m = slope.
Find the slope of the line of the graph given, using 2 points on the line, points (0, 2) and (1, 5).
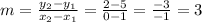
Substitute (x1, y1) = (1, 5) and m = 3 into
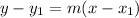
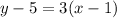
The answer is D.
Problem 2:
Slope-intercept equation takes the form:
 , where, m = slope, and b = y-intercept.
, where, m = slope, and b = y-intercept.
Find m and b.
Given, points (−4,−9) and (−2,−6),
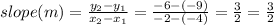
Substitute x = -2, y = -6, and m = ³/2 into
 , to find b:
, to find b:
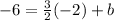 ,
,
Subtract b from both sides

Add 3 to both sides
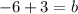

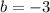
Substitute m = ³/2, b = -3 into

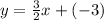
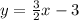
The answer is B.