Question

Find the distance (8,-7) (-4,-2)

Answer 1

`\boxed {d = 13}`

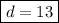
Explanation:

Use the Distance Formula to help you find the distance between the two following points:

`d = \sqrt{(x_(2) - x_(1))^(2) + (y_(2) - y_(1))^(2)}`

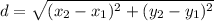
(where
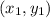`(x_(1), y_(1))` represents the first point and
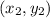`(x_(2), y_(2))` represents the second point)

-Apply the two following onto the formula:

`(x_(1), y_(1)) = (8, -7)`

`(x_(2), y_(2)) = (-4, -2)`

`d = \sqrt{(-4 - 8)^(2) + (-2 + 7)^(2)}`

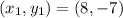
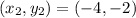
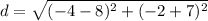
-Solve for the distance:

`d = \sqrt{(-4 - 8)^(2) + (-2 + 7)^(2)}`

`d = \sqrt{(-12)^(2) + 5^(2)}`

`d = √(144 + 25)`

`d = √(169)`

`\boxed {d = 13}`

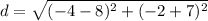
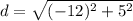
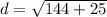
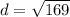
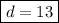
Therefore, the distance is
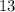`13`.

Answer 2

Distance = 13 units

Point A: (8, -7)

Point B: (-4, -2)

`\sqrt{(8 + 4)^(2) + (-7 + 2)^(2) }`

`\sqrt{(12)^(2) + (-5)^(2) }`

`√(144 + 25 )`

`√(169)`

`13`