Answer:
RT = 12 units
Explanation:
From the figure attached,
ΔSRQ is right triangle.
m∠R = 90°
An altitude has been constructed from point T to side SQ.
m∠RTQ = 90°
By applying geometric mean theorem in triangle SRQ,
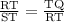

x² = 16 × 9
x² = 144
x = √144
x = 12
Therefore, length of altitude RT is 12 units.