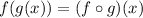Question

F(x) = x2 + 1
f(f(x))

Answer 1

This answer is for f(x) = 2x + 1:

f( f(x) ) = 4x + 3

Explanation:

If f(x) = 2x + 1, then f( f(x) ) is:

f(x) = 2x + 1

f( f(x) ) = 2( f(x) ) + 1

= 2 (2x + 1) + 1

= 4x + 2 + 1

f( f(x) ) = 4x + 3

Answer 2

Answer: f( f(x) ) = x^4 + 2x^2 + 2

=============================================

Work Shown:

f(x) = x^2 + 1

f(x) = ( x )^2 + 1

f( f(x) ) = ( f(x) )^2 + 1 .. replace every x with f(x)

f( f(x) ) = ( x^2+1 )^2 + 1 .. plug in f(x) = x^2+1

f( f(x) ) = ( x^2+1 )( x^2+1 )+1

f( f(x) ) = y( x^2+1 )+1 .... let y = f(x) = x^2+1

f( f(x) ) = y*x^2+y+1 ... distribute

f( f(x) ) = x^2*( y ) + ( y ) + 1

f( f(x) ) = x^2*( x^2+1 ) + (x^2+1) + 1 .... plug in y = x^2+1

f( f(x) ) = x^4 + x^2 + x^2 + 1 + 1

f( f(x) ) = x^4 + 2x^2 + 2

--------------------------------------

Side note:
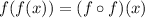`f(f(x)) = (f \circ f)(x)`, the small circle indicates function composition. It's similar to
`f(g(x)) = (f \circ g)(x)`