Question

A ladder 20 m long rests against a vertical wall so that the foot of the ladder is 9 m from the wall. a Find, correct to the nearest degree, the angle that the ladder makes with the wall. b Find, correct to 1 d.p., the height above the ground at which the upper end of the ladder touches the wall.​

Answer 1

(a) 27°

(b) 17.9m

Explanation:

According to the attachment

⠀

let AB be the ladder

BC be the distance between the ladder and wall

⠀

(a) We have to find ∠A by trigonometry formula

`\sf \sin A = (perpendicular)/(hypotenuse)`

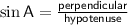
Here angle A is facing BC so it is perpendicular

and AB is the longest side so it is hypotenuse

`\sf \implies \sin A = (BC)/(AB) \\ \\ \\ \sf \implies \sin A = (9)/(20) \\ \\ \\ \sf \implies \sin A = 0.45 \\ \\ \\ \sf \implies \sin A = \sin27{ \degree} \\ \\ \\ \sf \purple {A = 27{ \degree}}`

(b)

AC is the distance from the upper end of the ladder to ground

we will find it by Pythagoras theorem

`\sf {hypotenuse}^(2) = {perpendicular}^(2) + {base}^(2) \\ \\ \\ \sf \implies {AB}^(2) = {BC}^(2) + {AC}^(2) \\ \\ \\ \sf \implies {20}^(2) = {9}^(2) + {AC}^(2) \\ \\ \\ \sf \implies 400 = 81 + {AC}^(2) \\ \\ \\ \sf \implies 400 - 81 = {AC}^(2) \\ \\ \\ \sf \implies 319 = {AC}^(2) \\ \\ \\ \sf \implies √(319) = AC \\ \\ \\ \sf \blue{\implies 17.86 = AC}`