Answer:
(a) 0.000061
(b) 0.000061
(c) 0.209
Explanation:
An array of 14 bits is equally likely to be 0 or 1.
That is, P (0) = P (1) = 0.50.
(a)
Compute the probability that all bits are 1s as follows:
![P(\text{All bits are 1s})=[P(1)]^(14)](https://img.qammunity.org/2021/formulas/mathematics/college/n3rzqtkbmsy97vtqg3phbzw31ktw0kta82.png) ∵ the bits are independent
∵ the bits are independent
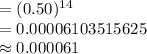
Thus, the probability that all bits are 1s is 0.000061.
(b)
Compute the probability that all bits are 0s as follows:
![P(\text{All bits are 0s})=[P(0)]^(14)](https://img.qammunity.org/2021/formulas/mathematics/college/lnm1h291b4yzeywmklybt9jx08w2uqeeeh.png) ∵ the bits are independent
∵ the bits are independent
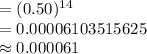
Thus, the probability that all bits are 0s is 0.000061.
(c)
Compute the probability that exactly 7 bits are 1s and 7 bits are 0s as follows:
Define X as the number of bits that 1s.
Then the random variable X will follows a binomial distribution with parameters n = 14 and p = 0.50.
The value of P (X = 7) is:
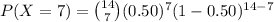

Thus, the probability that exactly 7 bits are 1s and 7 bits are 0s is 0.209.