Answer:
a)the weekly profit as a function of price is
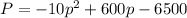
b) a bottle of wine be sold at $30 to realise maximum profit
c) the maximum profit that can be made by the producer is $2500
Explanation:
The weekly demand curve of a local wine producer is p= 50-0.1q
p = price
q = quantity
Revenue function R =Price \times Quantity
R=(50-0.1q)q
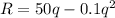
Cost function: c= 1500+ 10q
Profit function=R(x)-C(x)
Profit function=

Profit function=
 ----1
----1
We have q = 500 - 10 p using p = 50 − 0.1q

General quadratic equation:

On comparing
a = -0.1 , b = 40 , c = -1500
Maximum Profit is at q =
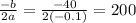
To find price must a bottle of wine be sold to realise maximum profit
p= 50-0.1q
p= 50-0.1(200)=30
Substitute the value of q in profit function(1) get the maximum profit
So, Profit function=
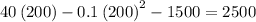
Hence
a)the weekly profit as a function of price is
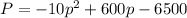
b) a bottle of wine be sold at $30 to realise maximum profit
c) the maximum profit that can be made by the producer is $2500