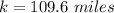Answer:
The value is
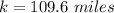
Explanation:
The diagram illustrating the question is shown on the first uploaded image
From the question we are told that
The distance from city A to B is AB = 467.3 miles
The bearing from B to C is
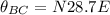
The bearing from B to A is
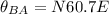
The bearing from A to B is
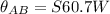
The bearing from A to C is
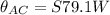
Generally from the diagram
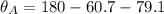
=>
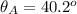
Also

and

=>
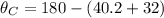
=>
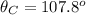
Generally according to Sine Rule
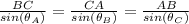
=>
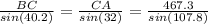
So
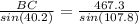
=>
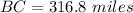
Also
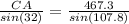
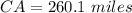
Generally the additional flyer miles that Adam will receive if he takes the connecting flight rather than the direct flight is mathematically represented as
![k = [CA +BC] - AB](https://img.qammunity.org/2021/formulas/mathematics/high-school/obmrl6ucnfrwojs49pemgq8gn7g6g7lh5k.png)
=>
![k = [260 .1 +316.8]- 467.3](https://img.qammunity.org/2021/formulas/mathematics/high-school/j3175a9mrlk15xizkvg0pp1xsldkkn45g5.png)
=>