Question

You drop your frozen rock from a green bridge. The frozen rock starts from rest (initial velocity = 0ms). The rock takes 4.3s to hit the water below. The acceleration due to gravity on Earth is 9.8m\s2 s 2 downward. What is the frozen rocks velocity after 1.5 seconds? vf = vi + at

Answer 1

The velocity of the frozen rock at
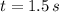`t = 1.5\,s` is -14.711 meters per second.

Step-by-step explanation:

The frozen rock experiments a free fall, which is a type of uniform accelerated motion due to gravity and air viscosity and earth's rotation effect are neglected. In this case, we need to find the final velocity (
`v`), measured in meters per second, of the frozen rock at given instant and whose kinematic formula is:

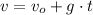`v = v_(o) + g\cdot t` (Eq. 1)

Where:

`v_(o)` - Initial velocity, measured in meters per second.

`g` - Gravity acceleration, measured in meters per square second.

`t` - Time, measured in seconds.

If we get that
`v_(o) = 0\,(m)/(s)`,
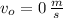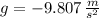`g = -9.807\,(m)/(s^(2))` and
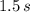`1.5\,s`, then final velocity is:

`v = 0\,(m)/(s)+\left(-9.807\,(m)/(s^(2)) \right) \cdot (1.5\,s)`

`v = -14.711\,(m)/(s)`

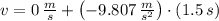
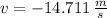
The velocity of the frozen rock at
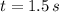`t = 1.5\,s` is -14.711 meters per second.