Answer:
a
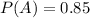
b

Explanation:
From the question we are told that
The percentage of ENC made by Alice is P(A) 85% = 0.85
The percentage of ENC made by Byte P(B) 10%=0.10
The percentage of ENC made by Cognizant P(C) = 5%= 0.05
The percentage rate of defect of ENC made by Alice is p = 2.5 % = 0.025
The percentage rate of defect of ENC made by Byte is q = 4.0% = 0.04
The percentage rate of defect of ENC made by Cognizant r = 6% =0.06
Generally the probability that a randomly selected ENC will be made by Alice is
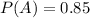
Generally the probability that a randomly selected ENC that was found to be defective will be made by Alice is
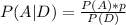
Here
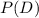 is the probability that a randomly selected ENC will be defective and this is mathematically represented as
is the probability that a randomly selected ENC will be defective and this is mathematically represented as
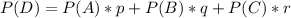
=>
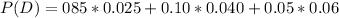
=>
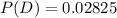
So
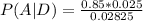
=>
