Answer:
(i) Final pressure and temperature are 25.119 bar and 753.566 K, (ii) Final pressure and temperature are 66.289 bar and 994.336 K.
Step-by-step explanation:
This system experiments an adiabatic compression, as such compression happens with no heat interaction between the piston-cylinder device and surroundings. This is a particular case of polytropic process, in which there is no entropy generation according to the Second Law of Thermodynamics.
The compression process is represented by the following formulas:
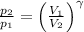 (Eq. 1)
(Eq. 1)
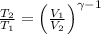 (Eq. 2)
(Eq. 2)
Where:
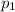 ,
,
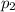 - Initial and final pressures, measured in bar.
- Initial and final pressures, measured in bar.
 ,
,
 - Initial and final temperatures, measured in Kelvins.
- Initial and final temperatures, measured in Kelvins.
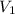 ,
,
 - Initial and final volumes, measured in cubic meters.
- Initial and final volumes, measured in cubic meters.
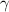 - Specific heat ratio of air, dimensionless.
- Specific heat ratio of air, dimensionless.
From Theory of Diesel and Otto Cycles we know that compression ratio is defined as:
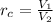 (Eq. 3)
(Eq. 3)
And (Eqs. 1, 2) can be rewritten as follows:
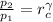 (Eq. 1b)
(Eq. 1b)
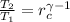 (Eq. 2b)
(Eq. 2b)
Then, we clear final pressure and pressure in each expression and calculate them for each case:
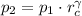
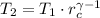
(i)
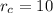 ,
,
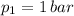 ,
,
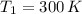 ,
,

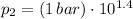
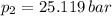
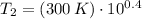
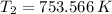
Final pressure and temperature are 25.119 bar and 753.566 K.
(ii)
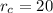 ,
,
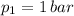 ,
,
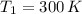 ,
,

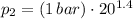


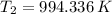
Final pressure and temperature are 66.289 bar and 994.336 K.