Given
The function is

The function g(x) transform by shifting f(x) right 3 units.
To find:
The function g(x).
Explanation:
The translation is defined as
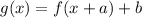
where, a is horizontal shift and b is vertical shift.
If a>0, then the graph shifts a units left and if a<0, then the graph shifts a units right.
If b>0, then the graph shifts b units up and if b<0, then the graph shifts b units down.
The function f(x) shifts only 3 units right. So,

Now,


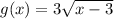
![[\because f(x)=3√(x)]](https://img.qammunity.org/2021/formulas/mathematics/college/rijo2gn0un4l8j5g2fx3m14kay8mjhvjyg.png)
Therefore, the required function is
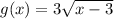 .
.