Answer:
AB = 75
BC = 60
AC = 45
m∠A = 53°
m∠B = 37°
m∠C = 90°
Explanation:
Trigonometric ratios

where:
 is the angle
is the angle- O is the side opposite the angle
- A is the side adjacent the angle
- H is the hypotenuse (the side opposite the right angle)
Given:
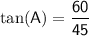
Therefore:
- side opposite angle A = BC = 60
- side adjacent angle A = AC = 45
To find the length of AB (the hypotenuse), use Pythagoras’ Theorem:

(where a and b are the legs, and c is the hypotenuse, of a right triangle)
⇒ AC² + BC² = AB²
⇒ 45² + 60² = AB²
⇒ AB² = 5625
⇒ AB = √5625
⇒ AB = 75
To find m∠A:
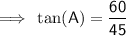
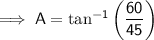

m∠C = 90° (as it is a right angle)
The interior angles of a triangle sum to 180°
⇒ m∠A + m∠B + m∠C = 180°
⇒ 53° + m∠B + 90° = 180°
⇒ m∠B = 180° - 53° - 90°
⇒ m∠B = 37°