Question

Write an equation of the line that passes through the point P(0,0) and is perpendicular to the line y=-5x+3

Answer 1

`y=(1)/(5)x`

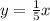
Explanation:

We want to write an equation of a line that passes through the point P(0, 0) and is perpendicular to the line y=-5x+3.

First, let's figure out the slope of our new line. Remember that slopes of perpendicular lines are negative reciprocals of each other.

So, to find our new slope, we need to flip our old slope and multiply by -1. So, our new slope is:

`-5`

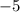
Flip:

`-(1)/(5)`

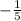
Multiply by a negative:

`=(1)/(5)`

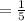
So, the slope of our new line is 1/5.

To find our equation, we can use the slope-intercept form:

`y=mx+b`

Where m is the slope and b is the y-intercept.

As previously determined, our slope is 1/5.

Note that (0, 0) is our y-intercept (and also our x-intercept). So, substitute 0 for b. Therefore, our new equation is:

`y=(1)/(5)x+0`

Simplify:

`y=(1)/(5)x`

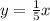
And we're done!