Answer:
The probability that neither test is positive
P(A⁻ ∩ B⁻ ) = 0.0108
Explanation:
Step(i) :-
Given the two tests are independently
Test A has probability 0.91 of being positive if the illegal drug has been used
P(A) = 0.91
Test B has probability 0.88 of being positive if the illegal drug has been used
P(B) = 0.88
If events are independently then ,
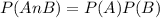
Step(ii):-
The probability that neither test is positive (and thus Al gets to keep his job)

P(A⁻ ∩ B⁻ ) = P(A⁻ ) P(B⁻ )
= ( 1- P(A) ) ( 1 - P(B))
= (1 - 0.91 ) ( 1 - 0.88 )
= (0.09) (0.12)
= 0.0108
The probability that neither test is positive
P(A⁻ ∩ B⁻ ) = 0.0108