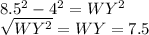Question

In the figure below, the segments WX and W Y are tangent to the circle centered at 0. Given that OX= 4 and OW = 8.5, find WY.

Answer 1

7.5

Explanation:

because OY is a radius like OX they will have equal length of 4 so OY = 4

Because of the circle theorem that says that tangents meet the the radius of a circle at 90 degrees, angle OYW is 90 degrees so the triangle OYW is a right angle triangle and we can use pythagoras theorem to get WY as:

`8.5^(2) - 4^(2) = WY^(2) \\\sqrt{WY^(2)}=WY=7.5`