Question

HELP!!

Which of the statements below are true for linear functions? Select all that apply.

1. The general equation is y = mx + b.

2. The general equation is y = ax^2 + bx + c

3. The general equation is y = ab^x

4. The graph contains a vertex

5. The slope of the graph is constant and can be defined as rise over run.

Answer 1

`\rule{60}{1}\:\:\:\rule{60}{1}\:\:\:\rule{60}{1}\:\:\:\rule{60}{1}`

`\diamond\large\blue\textsf{\textbf{Given question:-}}}`

Which of the statements below are true for linear functions?

Select all that apply.

`\diamond\large\blue\textsf{\textbf{\underline{\underline{Answer and how to solve:-}}}}`

The general equation for linear functions is
`\bold{y=mx+b}`.

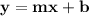The equation
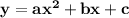`\bold{y=ax^2+bx+c}` is the general equation for quadratic functions.

The equation
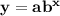`\bold{y=ab^x}` is the general equation for exponential functions.

Linear functions don't have a vertex, they have slope (m) and y-intercept (b).

One way we can define the slope of a linear function is

`\bold{Slope=(Rise)/(Run)}`

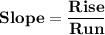
So we conclude that the right options are

`\square\!\!\!\!\checkmark` The general equation is y=mx+b.

`\square\!\!\!\!\checkmark` The slope of the graph is constant and can be defined as rise over run.

Good luck with your studies.

`\rule{300}{1}`