Question

Why aren’t the following polynomials? y=x^2 +2^x y^2=(x-2)^2-1 y=1/x^2+1/x+1/2

Answer 1

Polynomials can have constants (3, -9), variables (x, y), and exponents (
`x^(2)`). One thing you can't have is a variable in the denominator. For example:
`(2)/(x+3)`

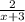
Or fractional exponents.

Explanation:

a)
`y=x^(2) +2^(x)`

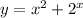
Is not a polynomial because
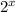`2^(x)` does not have the standard form, where variable is the base. e.g.
`x^(2)`

b)
`y^(2)=(x-2)^(2)-1`

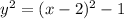
Is not a polynomial because
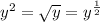`y^(2) =√(y) =y^{(1)/(2) }` has fractional exponents

c)
`y=(1)/(x^(2) ) +(1)/(x+(1)/(2) )`

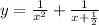
Is not a polynomial because our variable x is in the denominator