Answer:
The answer is
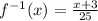
Explanation:
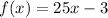
To find the inverse of f(x) , equate f(x) to y
That's
y = f(x)
We have
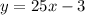
Next interchange the terms that's x becomes y and y becomes x
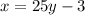
Next solve for y
Move 3 to the other side of the equation
That's
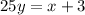
Divide both sides by 25
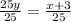
We have the final answer as
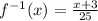
Hope this helps you