Answer:
The value is

Step-by-step explanation:
From the question we are told that
The number of students is
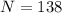
The metabolic rate for each student is
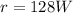
The time duration is

The molar specific heat of air is
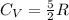
The volume is
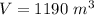
The pressure is
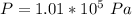
The initial temperature is
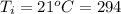
Generally the metabolic rate of the students is
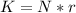
=>
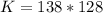
=>
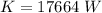
The total heat generated by the students is
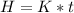
=>
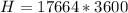
=>
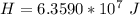
From the ideal gas law we can evaluate n (number of moles ) as
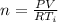
Here R is the gas constant with value
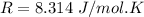
So
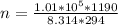
=>
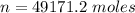
Generally the heat generated is mathematically represented as

=>
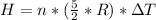
=>
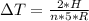
=>
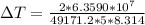
=>
