Question

In Nebraska, the mean rain-fed corn yield of harvested fields are normally

distributed, produce 147.2 bushels per acre with a standard deviation of 33 bushels per acre.
How many bushels per acre are produced if a field is in the top 25%?
O 180.2
O 125.2
O 155.45
O 169.2

Answer 1

Answer: 169.2 per acre are produced if a field is in the top 25%.

Explanation:

Given: The mean rain-fed corn yield of harvested fields are normally distributed, produce 147.2 bushels per acre with a standard deviation of 33 bushels per acre.

Let X denote the mean rain-fed corn yield of harvested fields.

Let x denotes the number of bushels per acre are produced if a field is in the top 25%.

Top 25% on a density curve means , (100-25%)i.e. 75% area lies below x.

I.e.

`P(X<x)=P((X-\mu)/(\sigma)<(x-147.2)/(33))=0.75\\\\\Rightarrow\ P(Z<(x-147.2)/(33))=0.75\ \ [Z=(X-\mu)/(\sigma)]`.

Z-score for p-value of 0.75 (one tailed)=0.67 [By z-table]

Then,
`(x-147.2)/(33)=0.6744`

`\Rightarrow\ {x-147.2}=33*0.67\\\\\Rightarrow\ {x-147.2}=22.11\\\\\Rightarrow\ {x}=22.11+147.2=169.31`

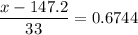
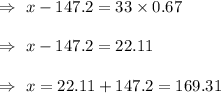
Here approx value is 169.2 (from options).

So, 169.2 per acre are produced if a field is in the top 25%.