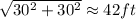Question

A guy wire supports an antenna tower, as shown at the right. The bottom of the wire is secured in the ground 30 feet from the base of the tower. The top of the wire is secured to the tower at a height of 30 feet above the ground. How long is the wire? Round your answer to the nearest tenth of a foot.

Answer 1

The wire is approximately 42feet long

Explanation:

We can mentally sketch out the shape that is formed between the guy wire and the antenna tower. This is simply a right-angled triangle with the opposite side being the height of the antenna (30 feet). The adjacent side is the distance on the ground between the point where the wire was fastened and the base of the antenna.

The hypotenuse side is the length of the wire we are looking for.

Parameters are given as:

Opposite : 30ft

Adjacent: 30ft

Hypotenuse: x feet

The hypotenuse =
`√(opp^2 + adj^2)`

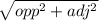
Hypotenuse =
`√(30^2+ 30^2) \approx 42ft`