Question

Find the relative extrema of the function, if they exist. Also, state the intervals where the function is increasing or decreasing. g

Answer 1

The question is not complete. I will explain relative extrema and how to calculate it.

Explanation:

The singular of extrema is extremum and it is simply used to describe a value that is a minimum or a maximum of all function values.

A function will have relative extrema (relative maximum or relative minimum) at points in which it changes from decreasing to increasing, or vice versa.

So if f(y) is a function of y

• Function f(d) will be is a relative maximum of f(y),

if there exists an interval (a, b) containing d

such that for all y in (a, b) , f(y) ≤ f(d)

• Function f(d) will also be a relative minimum of f(y),

if there exists an interval (a, b) containing d

such that for all y in (a, b) , f(y) ≥ f(d)

Kindly note that If f(d) is a relative extrema of f(y), then the relative extrema occurs at y = d.

For the local extrema of a critical point to be determined, the function must go from increasing, that means positive
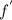`f^(')`, to decreasing, that means negative
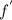`f^(')`, or vice versa, around that point.

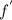`f^(')` is determined by finding the first derivative of the function f(y). The relative extrema will therefore allows us to check for any sign changes of f′ around the function's critical points.