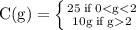Question

Piecewise Function - The domain is split

Answer 1

The piecewise function would be as follows :

`\mathrm{C(g) = \left \{ {{25\:if\: 0 < g < 2} \atop {10g\:if\:g>2}} \right. }`

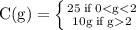
Explanation:

This piecewise function is composed of one segment, and a ray. Let's start by identifying the properties of this segment.

Segment : As you can see the segment extends from 0 to 2 on the x - axis. This is at y = 25. Therefore our first expression would be 'C(g) = 25 at {0 < g < 2}.'

Ray : As this is ray, we have C(g) = 10g, as the slope is apparently 10. As you can see the rise is 10, over a run of 1, given the points (2, 25) and (3, 35) lie on the plane. The ray starts at the coordinate (2, 25), leaving us with the inequality g > 2.

So now that we have the expressions 'C(g) = 25 at {0 < g < 2}' and 'C(g) = 10g at {g > 2}' we can combine them to create the following piecewise function,

`\mathrm{C(g) = \left \{ {{25\:if\: 0 < g < 2} \atop {10g\:if\:g>2}} \right. }`