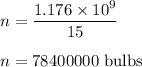Step-by-step explanation:
It is given that,
Water flows over Niagara Falls at the average rate of 2,400,000 kg/s
The average height of the falls is 50 m
We need to find the power of Niagara Falls.
The gravitational potential energy of falling water is given by :
P = mgh
Power is rate of doing work i.e.
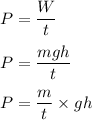
We have, m/t = 2,400,000 kg/s
So,
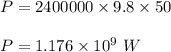
If the number of bulbs are n that could power 15 W LED, the,