Question

A wheel that is rotating at 33.3 rad/s is given an angular acceleration of 2.15 rad/s 2. Through what angle has the wheel turned when its angular speed reaches 72.0 rad/s

Answer 1

The angle through which the wheel turned is 947.7 rad.

Step-by-step explanation:

initial angular velocity,
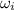`\omega _i` = 33.3 rad/s

angular acceleration, α = 2.15 rad/s²

final angular velocity,
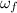`\omega_f` = 72 rad/s

angle the wheel turned, θ = ?

The angle through which the wheel turned can be calculated by applying the following kinematic equation;

`\omega_f^2 = \omega_i^2 + 2\alpha \theta\\\\\theta = (\omega_f^2\ -\ \omega_i^2)/(2\alpha ) \\\\\theta = ((72)^2\ -\ (33.3)^2)/(2(2.15))\\\\\theta = 947.7 \ rad`

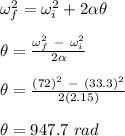
Therefore, the angle through which the wheel turned is 947.7 rad.