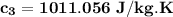Answer:
The specific heat of the sample
Step-by-step explanation:
Given that:
mass of an unknown sample
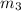 = 0.0850
= 0.0850
temperature of the unknown sample
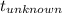 = 100° C
= 100° C
initial temperature of the calorimeter can = 19° C
mass of copper
 = 0.150 kg
= 0.150 kg
mass of water
 = 0.20 kg
= 0.20 kg
the final temperature of the calorimeter can = 26.1° C
The objective is to compute the specific heat of the sample.
By applying the principle of conservation of energy

where;
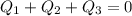
i.e
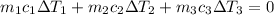
the specific heat capacities of water and copper are 4.18 × 10³ J/kg.K and 0.39 × 10³ J/kg.K respectively
the specific heat of the sample
 can be computed by making
can be computed by making
 the subject of the above formula:
the subject of the above formula:
i.e
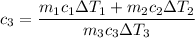
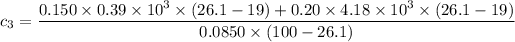
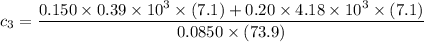
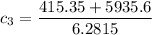
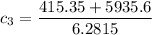

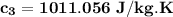
The specific heat of the sample