Question

An artist has a block of clay in the shape of a cube. The edges of the cube measure 3 inches. The clay will be used to make solid cones with a base diameter of 1.5 inches and a height of 2 inches. How many cones can be made

Answer 1

n = 23

Explanation:

Given that,

The edge of the cube is 3 inches

The diameter of a solid cone is 1.5 inches

Height of the cone is 2 inches

We need to find the number of cones that can be made from the block of clay which is in the shape of a cube. Let there are n such cones. So,

`n=\frac{\text{volume of cube}}{\text{volume of a cone}}\\\\n=(l^3)/((1)/(3)\pi r^2 h)`

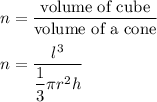
l is side of a cube

So,

`n=((3)^3)/((1)/(3)* (22)/(7)* ((1.5)/(2))^2* 2)\\\\n=22.9`

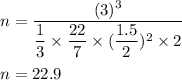
or

n = 23 approx

So, 23 cone can be made.