Question

Find the average rate of change of f(x) with respect to x on the interval.
`f(x)=x^2-4 ; [-4,3]`

Answer 1

The average rate of function of f on the given interval is -1.

Explanation:

Recall that the average rate of change is simply the slope of the function between two points.

We are given the function:

`f(x)=x^2-4`

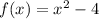
And we want to find its average rate of change on the interval [-4, 3].

Evaluate the two endpoints:

`\displaystyle \begin{aligned} f(-4) & = (-4)^2 - 4 \\ \\ & = 16 - 4 \\ \\ & = 12\end{aligned}`

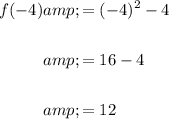
Likewise:

`\displaystyle \begin{aligned} f(3) & = (3)^2 - 4 \\ \\ & = 9 - 4 \\ \\ & = 5\end{aligned}`

Recall that slope is given by:

`\displaystyle m = (\Delta y)/(\Delta x)`

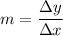
Hence, the average rate of change is:

`\displaystyle\begin{aligned} \text{Avg Rate of Change} & = ((5)-(12))/((3)-(-4)) \\ \\ &= (-7)/(7) \\ \\ & = -1\end{aligned}`

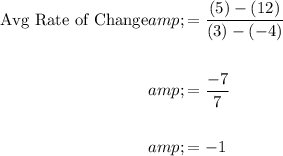
In conclusion, the average rate of function of the given function on the given interval is -