Question

If $-3\le x+5 \le 8$ and $x$ is an integer, what is the sum of all the possible solutions?

Answer 1

-30

Explanation:

The integers are the whole numbers and the opposite of the whole numbers.

That is {...,-3,-2,-1,0,1,2,3,...} is the set of integers.

If it is a number you count with or the opposite of a number you count with it or 0, then it is an integer.

So we need to solve the inequality
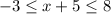`-3 \le x+5 \le 8` first, and then just say all the integers included in that compound inequality. Last step is to add since it is asking for the sum of those integers.

Let's do this.

`-3 \le x+5 \le 8`

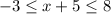
Subtract 5 on all sides:

`-3-5 \le x+5-5 \le 8-5`

Simplify all sides:

`-8 \le x+0 \le 3`

`-8 \le x \le 3`

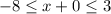
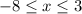
This inequality includes all numbers between -8 and 3, and both include -8 and 3 because of the equal part on both of the inequality parts.

So we have -8,-7,-6,-5,-4,-3,-2,-1,0,1,2, and 3 are the integer solutions of this inequality.

Let's add them. I'm going to put opposites together because I know they will zero out.

So I have this:

-8+-7+-6+-5+-4+-3+-2+-1+0+1+2+3

-8+-7+-6+-5+-4+(-3+3)+(-2+2)+(-1+1)+0

-8+-7+-6+-5+-4+0 +0 +0 +0

-8+-7+-6+-5+-4

-(8+7+6+5+4)

-([8+7]+[6+5]+4)

-([15]+[11]+4)

-(15+11+4)

-([15+11]+4)

-([26]+4)

-(26+4)

-(30)

-30