Question

M44b

4. In little league baseball, the distance of the paths between each pair of consecutive bases is 60 feet
and the paths form right angles. How far does the ball need to travel if it is thrown from home plate
directly to second base?

Answer 1

84.85 feet or 60
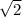`\sqrt2` feet.

Explanation:

Given that:

Distance of paths between each pair of consecutive bases is 60 ft.

To find:

Distance that the ball needs to travel if it is thrown directly from home plate to second base?

Solution:

Actually the given problem can be mapped to a right angled triangle in which base and height are given to be equal to 60 ft (because each pair of consecutive bases are 60 ft apart), Plus, they are at right angles to each other.

So, kindly refer to the image attached.

AB and BC are the distance of paths between consecutive bases.

And we have to find AC.

We can use Pythagorean theorem to find out the Hypotenuse of the triangle.

According to Pythagorean theorem:

`\text{Hypotenuse}^(2) = \text{Base}^(2) + \text{Perpendicular}^(2)\\\Rightarrow AC^(2) = AB^(2) + BC^(2) \\\Rightarrow AC^(2) = 60^(2) + 60^(2) \\\Rightarrow AC = √(2 * 60^2)\\\Rightarrow AC = \bold{60√(2)\ ft = 84.85\ ft}`