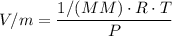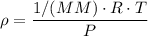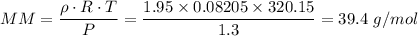Question

the density of a gas was measure at 1.30 atm and 470c and found to be 1.95 g/L.Calculate the molar mass of the gas. This is a Ap question can anyone help .

Answer 1

The molar mass = 39.4 g/mol

Step-by-step explanation:

The given parameters are;

The pressure at which the gas was measured = 1.3 atm.

The temperature of the gas = 47°C

The measured density of the gas = 1.95 g/L

By the combined gas equation, we have;

`P \cdot V = n \cdot R \cdot T`

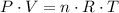
Where;

n = Number of moles = m/MM

P = Pressure = 1.3 atm

V = Specific, Molar Volume

T = Temperature = 47 °C = 320.15 K

R = Universal Gas Constant = 0.08205 L·atm/(mol·K)

ρ = The density = MM/V

Where;

MM = Molar mass

Therefore, V = MM×ρ

`V/m= (1/(MM) \cdot R \cdot T)/(P)`

`\rho= (1/(MM) \cdot R \cdot T)/(P)`

`MM = (\rho \cdot R \cdot T)/(P) =\frac{ 1.95 * 0.08205 * 320.15} {1.3} = 39.4 \ g/mol`