Question

If 1.7 kg of 238Pu is required to power the spacecraft's data transmitter, for how long after launch would scientists be able to receive data? Round to the nearest year. Do not round intermediate calculations.

Answer 1

The question is incomplete. Here is the complete question.

The isotope of Plutonium 238Pu is used to make thermoeletric power sources for spacecraft. Suppose that a space probe was launched in 2012 with 3.5 kg of 238Pu.

(a) If the half-life of 238Pu is 87.7 yr, write a function of the form
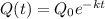`Q(t)=Q_(0)e^(-kt)` to model the quantity Q(t) of 238Pu left after t years. Round ythe value of k to 3 decimal places. Do not round intermediate calculations.

(b) If 1.7kg of 238Pu is required to power the spacecraft's data transmitter, for low long after launch would scientists be able to receive data? Round to the nearest year. Do not round intermediate calculations.

Answer: (a)
`Q(t)=3.5e^(-0.0079t)`

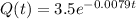
(b) 91 years.

Step-by-step explanation:

(a) Half-life is time it takes a substance to decrease to half of itself, i.e.:

Q(t) =
`0.5Q_(0)`

`0.5Q_(0)=Q_(0)e^(-87.7k)`

`0.5=e^(-87.7k)`

`ln(0.5)=ln(e^(-87.7k))`

`ln(0.5)=-87.7k`

`k = (ln(0.5))/(-87.7)`

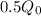
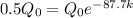
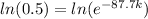
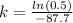
k = 0.0079

Knowing k and
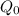`Q_(0)`=3.5kg, function is
`Q(t)=3.5e^(-0.0079t)`

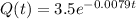
(b) Using function:

`Q(t)=3.5e^(-0.0079t)`

`1.7=3.5e^(-0.0079t)`

`e^(-0.0079t)=(1.7)/(3.5)`

`e^(-0.0079t)=0.4857`

`ln(e^(-0.0079t))=ln(0.4857)`

`-0.0079t=-0.7221`

`t = (-0.7221)/(-0.0079)`

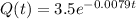
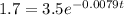
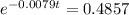
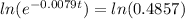
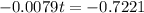
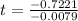
t = 91.41

t ≈ 91 years

Scientists will be able to receive data for approximately 91 years.