Question

Find the sum of 1 + 3/2 + 9/4 + …, if it exists. This is infinite series notation. The answer is NOT 4.75.

Answer 1

No Sum --- it doesn't exist.

Explanation:

The partial sums get arbitrarily large--the go to infinity.

The geometric series you are trying to sum has common ratio = 3/2.

The sum of the infinite series exists only when |common ratio| < 1.

The formula for the partial sum of n terms is (r^(n+1) - 1) / (r - 1) = (1.5^(n+1) - 1) / 0.5, or in decimals instead of fraction.. i.e. 1 + 1.5 + 2.25 + 5.0525 + 25.628 + 656.840..... therefore It would take a long time but you'd be adding up forever and goes to infinity.

Answer 2

D

Explanation:

First, this looks like a geometric series. To determine whether or not it is, find the common ratio. To do this, we can divide the second term and the first term, and then divide the third term and the second term. If they equal to same, then this is indeed a geometric series.

`(3/2)/(1)=3/2\\(9/4)/(3/2)=(9/4)(2/3)=18/12=3/2`

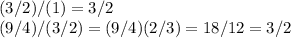
Therefore, this is indeed a geometric series with a common ratio of 3/2.

With just this, we can stop. This is because since the common ratio is greater than one, each subsequent value is going to be bigger than the previous one. Because of this, the series will not converge. Therefore, the series has no sum.

To see this more clearly, imagine a few more terms:

1, 1.5, 2.25, 3.375, 5.0625...

Each subsequent term will just increase. The sum will not converge.