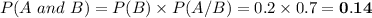Answer:
1) a) 0.8
b) 0.6
2) a) 0.08
b) 0.14
Explanation:
1) Given
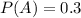 and
and

Let us learn about a formula:
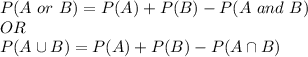
(a) If A and B are mutually exclusive i.e. no common thing in the two events.
In other words:
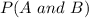 =
=
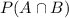 = 0
= 0
Using above formula:
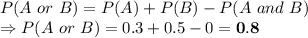
(b) P(A and B) = 0.2
Using above formula:

*************************************
1) Given
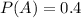 and
and
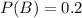
Let us learn about a formula:
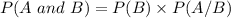 for dependent events
for dependent events
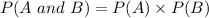 for independent events.
for independent events.
(a) If A and B are independent events :
Using the above formula for independent events:
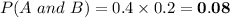
(b)
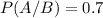
Using above formula: