Answer:
The radius of the black hole will be 2949.6 m.
Step-by-step explanation:
The radius of this black hole will be the Schwarzschild radius of the mass of the sun
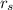 =
=
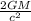
where
G is the gravitational constant = 6.67 x 10^-11 m^3⋅kg^-1⋅s^-2
M is the mass of the sun = 1.99×10^30 kg
c is the speed of light = 3 x 10^8 m/s
substituting values into the equation, we have
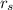 =
=
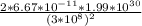 = 2949.6 m
= 2949.6 m