Complete Question
The complete question is shown on the first uploaded image
Answer:
a
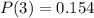
b

c
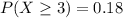
d
option C is correct
Explanation:
From the question we are told that
The probability of success is p = 0.4
The sample size is n= 4
This adults believe follow a binomial distribution is because there are only two outcome one is an adult believes in reincarnation and the second an adult does not believe in reincarnation
The probability of failure is mathematically evaluated as
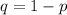
substituting values
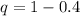

Considering a
The probability that exactly 3 of the selected adults believe in reincarnation is mathematically represented as
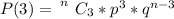
substituting values
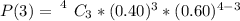
Here
 means 4 combination 3 . i have calculated this using a calculator and the value is
means 4 combination 3 . i have calculated this using a calculator and the value is
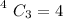
So
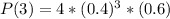
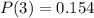
Considering b
The probability that all of the selected adults believe in reincarnation is mathematically represented as
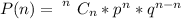
substituting values
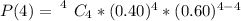
Here
 means 4 combination . i have calculated this using a calculator and the value is
means 4 combination . i have calculated this using a calculator and the value is

so

=>

Considering c
the probability that at least 3 of the selected adults believe in reincarnation is mathematically represented as
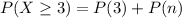
substituting values
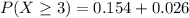
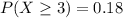
From the calculation the probability that all the 4 randomly selected persons believe in reincarnation is
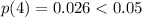
But the the probability of 3 out of the 4 randomly selected person believing in reincarnation is
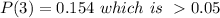
Hence 3 is not a significantly high number of adults who believe in reincarnation because the probability that 3 or more of the selected adults believe in reincarnation is greater than 0.05.