Answer:
a. See Attachment 1
b.
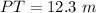
c.
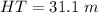
d.
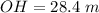
Explanation:
Calculating PT
To calculate PT, we need to get distance OT and OP
Calculating OT;
We have to consider angle 50, distance OH and distance OT
The relationship between these parameters is;
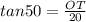
Multiply both sides by 20
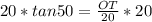
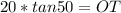
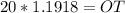
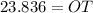

Calculating OP;
We have to consider angle 30, distance OH and distance OP
The relationship between these parameters is;
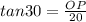
Multiply both sides by 20



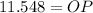
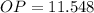
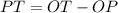
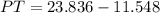
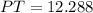
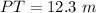 (Approximated)
(Approximated)
--------------------------------------------------------
Calculating the distance between H and the top of the tower
This is represented by HT
HT can be calculated using Pythagoras theorem
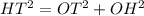
Substitute 20 for OH and

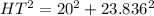
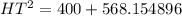
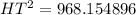
Take Square Root of both sides
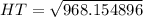
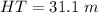 (Approximated)
(Approximated)
--------------------------------------------------------
Calculating the position of H
This is represented by OH
See Attachment 2
We have to consider angle 50, distance OH and distance OT
The relationship between these parameters is;
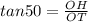
Multiply both sides by OT
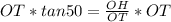


Substitute

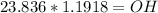
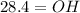
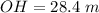 (Approximated)
(Approximated)